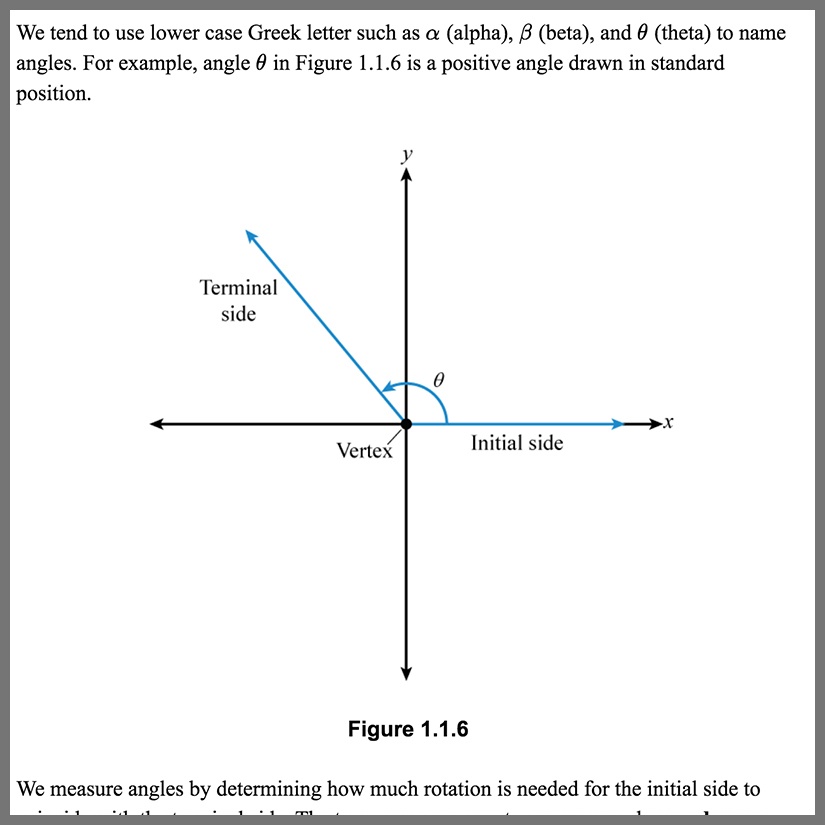
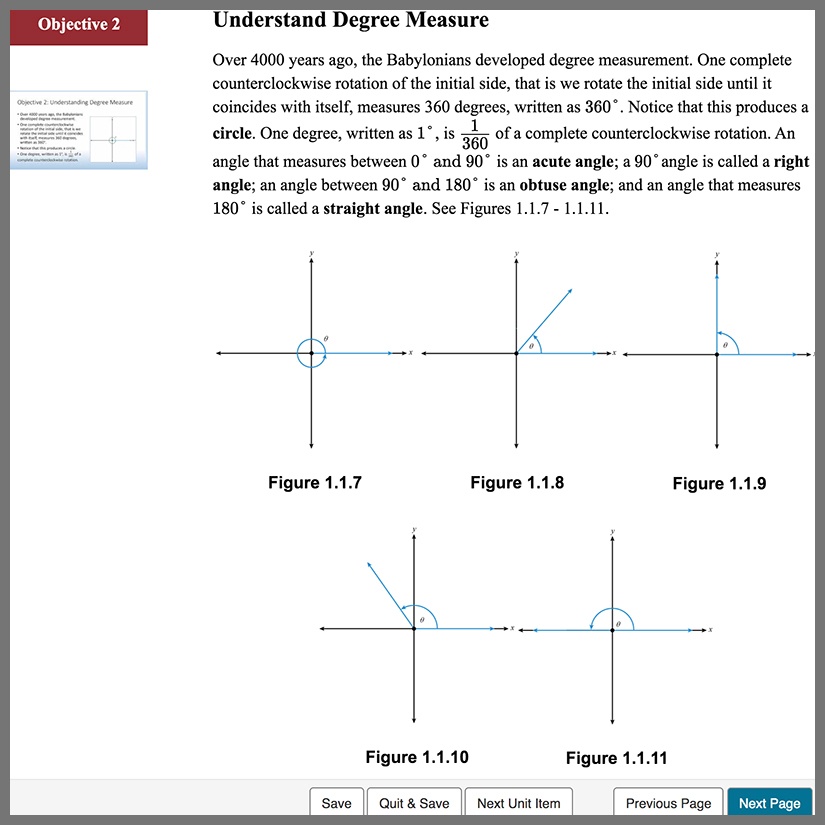
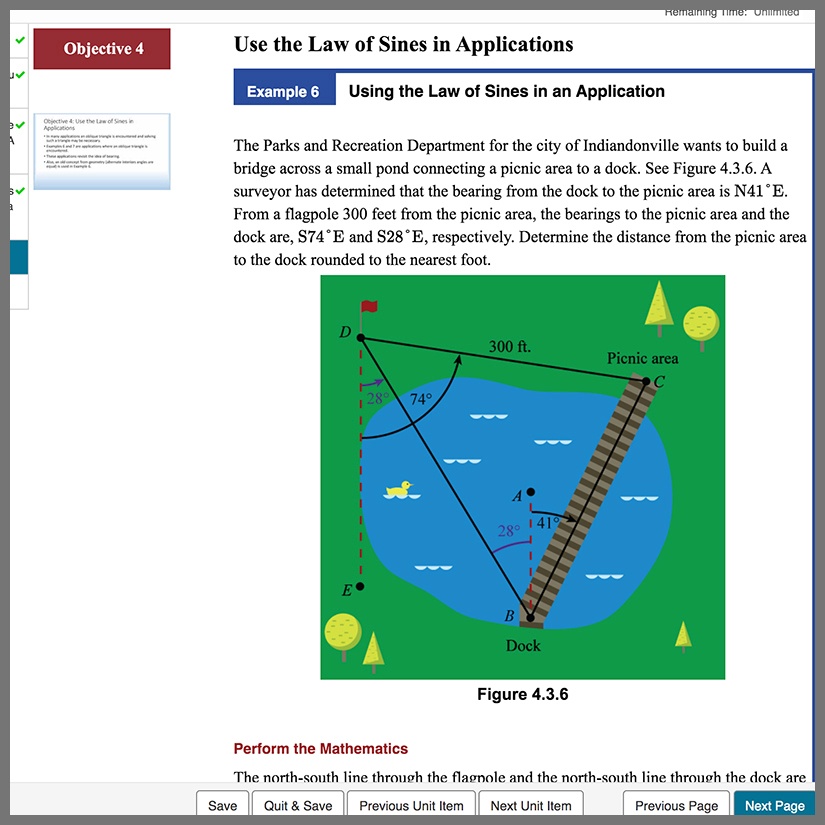
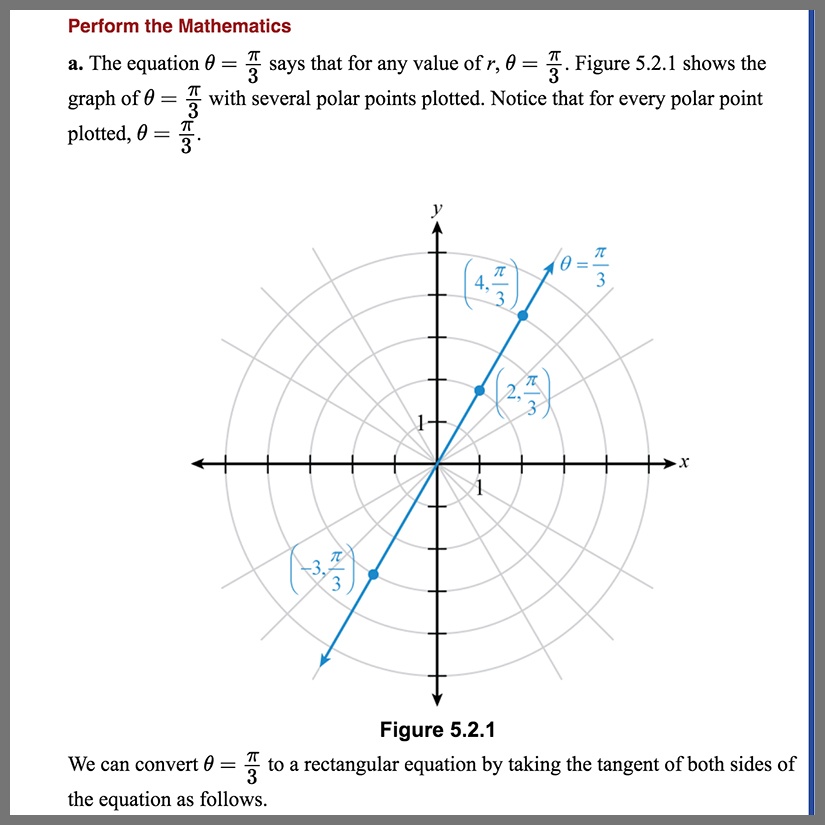
Description
The Trigonometry: A Unit Circle Approach that prepares for Calculus Content Pack is a trigonometry course presented using the unit circle approach that you can use as a customizable starting point to a complete college course in Möbius. This unit circle approach fits much better with the concept of functions. In addition, this approach allows most topics in trigonometry to be built upon the unit circle. This customizable resource contains 5 units of sectioned lessons and assignments enhanced with Möbius capabilities including algorithmic questions, embedded videos, and end-of-lesson quizzes. With a clear progression of concepts, it, along with our other text College Algebra: A Function Approach that prepares for Calculus, equip learners with the necessary tools to succeed in calculus and beyond.
How does Möbius take this Content Pack to the next level?
Learn how Möbius’ unique STEM question types throughout this content provide the best STEM learning experience.
Work with over 50 configurable assessment properties when modifying existing or building your own assessments.
Lessons help to solidify difficult STEM concepts and can be modified with Möbius’ editing tools to include additional Active Learning principles.
Watch the webinar from the authors of this content pack, “Creating A Student-Centric Textbook: Preparing Students for Calculus.”